<Informal because I have no energy, also partially for my own reference later. I’ve been busy when not blogging.>
The PCBs I designed for the Bluetooth biofeedback thing arrived a few days ago. From Oshpark, these were ~10usd total, with free shipping and took ~2 weeks. I’ve never done any QFN soldering (the leads are entirely under the IC) and I didn’t get a solderpaste stencil. Big mistake. Spent a lot of painstaking time with an Exacto knife, sewing needle and tweezers. Also tried using a hot air station for the first time, but a pan on the stove ended up working better.
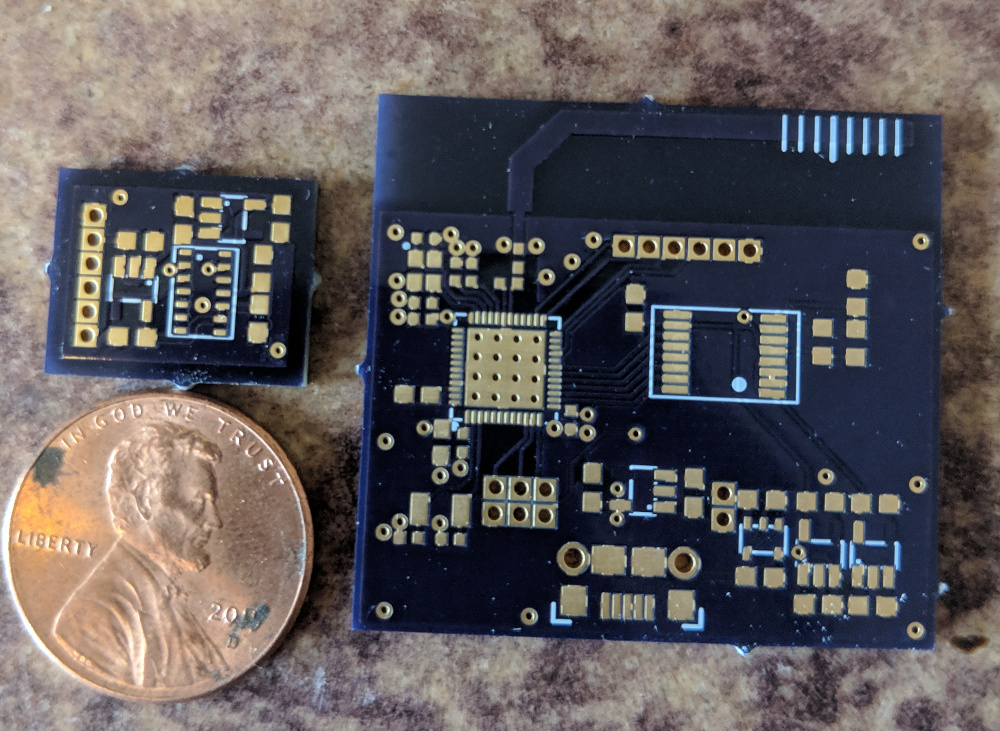
Small one is meant to be worn on a strap on the finger, the large one is meant to be worn on a strap on the wrist.
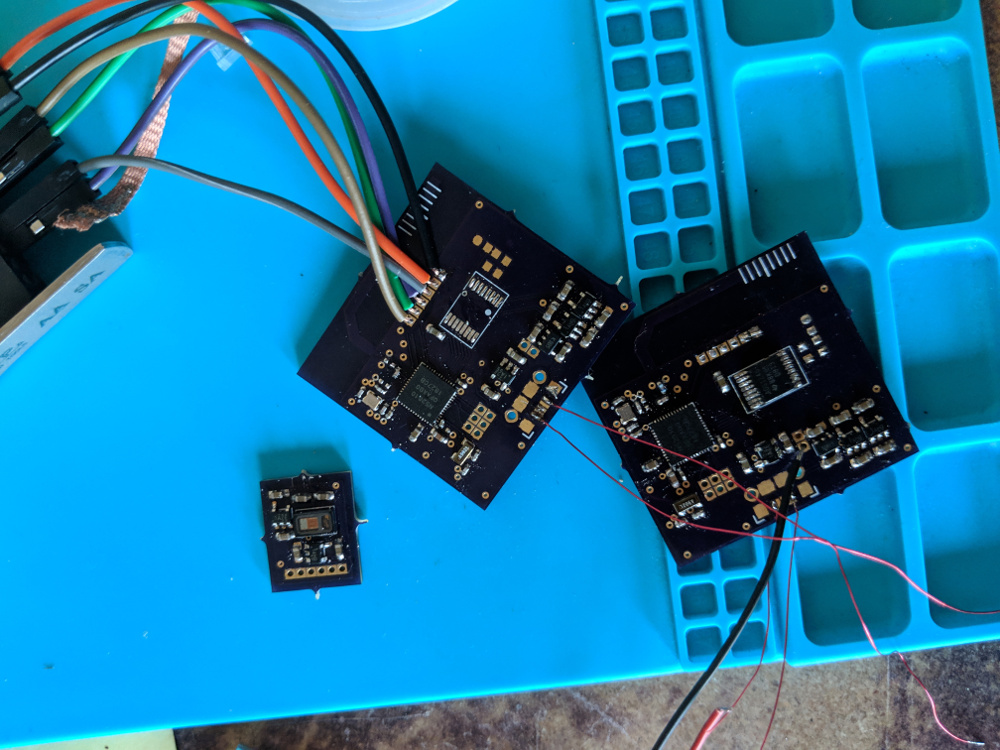
Anyway, I got 2 assembled and one of them works with my st-link and openocd. Unfortunately I lost all of my rf inductors during assembly (also the only parts I lost), so I can’t test the bluetooth. I’m using the nRF52810, have downloaded the SDK kit from https://www.nordicsemi.com/Software-and-Tools/Software/nRF5-SDK/Download#infotabs, and can get the examples to compile with the compiler from https://developer.arm.com/tools-and-software/open-source-software/developer-tools/gnu-toolchain/gnu-rm. I’m mentioning where I got the compiler because I spent a few hours trying to get the gentoo package crossdev working before just downloading binaries.
openocd -f ~/openocd/tcl/interface/stlink.cfg -f ~/openocd/tcl/target/nrf52.cfg -c “program nrf52810_xxaa.bin verify reset exit”
Because it will take time anyway for new inductors to arrive, I did a redesign and ordered more boards. I hope to actually be able to wear the next generation around. I’ll put in some filler posts on computing before then.
